Computing Derivatives Resources
Best of the Web. Picked by our PhDs
Websites
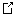
When it comes to basic derivatives, this is the last table you’ll ever need. Instead of deriving them using the tedious limit formula, you can just look them up here.
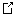
If you ever forget low-dee-high minus high-dee-low over low-low, you can always look up the quotient rule in a less phonetically terrifying form.
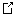
The chain rule isn’t a leash that your teacher uses to train you. Here are some examples that demonstrate how to use the chain rule.
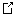
Like a shape-shifter constantly changing forms, the chain rule can be applied in several different notations. Learn how the chain rule is applied in all notations.
Videos
The chain rule is a powerful, time-saving tool. It’s the calculus equivalent of using a jack-hammer to break concrete over using a hammer and chisel. This video shows you why.
High-low, high-low, to the quotient rule we go. With low-dee-high minus high-dee-low, divide by low-low and a new derivative you will know.
The product rule isn’t a guide on how to increase your sales of purple desk chairs. It’s an easy-to-use rule to simplify complicated problems involving derivatives. If you pay close attention in this video, you’ll find an easy way to avoid using the quotient rule by using the product rule instead.
Yeah, we know. Implicit differentiation is terrible. You just wish it’d come out and explicitly ask you on a date. It’s so wrapped up its own little equation that it can’t. You’ll learn how to derive the derivative from it implicitly here.
Games and Tools
Sometimes, the quotient rule can leave you with a mess of numbers and letters floating around like an alphanumeric soup. Here’s a calculator to compute derivatives using the quotient rule, with step by step explanations. We hope it tastes as good as tomato soup once you figure out what you have left on paper.
This calculator will give you a way to explicitly check the implicit derivative of your equation. Pretty cool. Soon, computers will be able to fetch balls for you.