ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Algebra and Functions Videos 140 videos
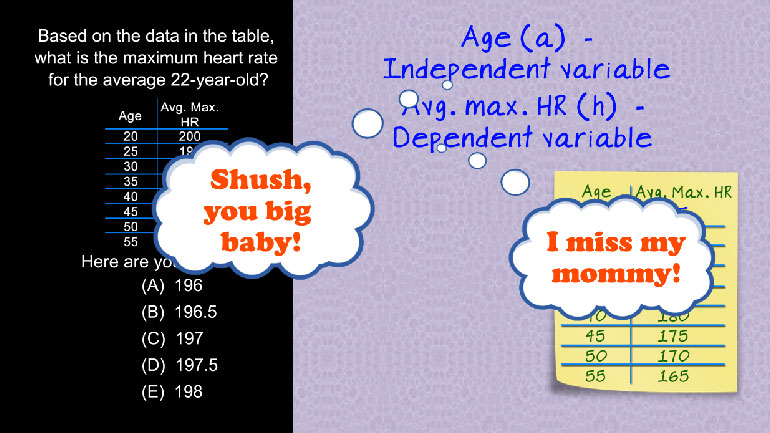
SAT Math: Algebra and Functions Drill 1, Problem 2. Based on the data in the table, what is the maximum heart rate for the average 22-year-old?
SAT Math 1.3 Algebra and Functions. How many 16 oz. bottles did she buy?
SAT Math 4.5 Algebra and Functions 197 Views
Share It!
Description:
SAT Math 4.5 Algebra and Functions
SAT Math 4.5 Algebra and Functions
Transcript
- 00:02
Here’s your shmoop du jour, brought to you by Dollar Signs.
- 00:05
Why can’t there be more of THOSE on the highway?
- 00:08
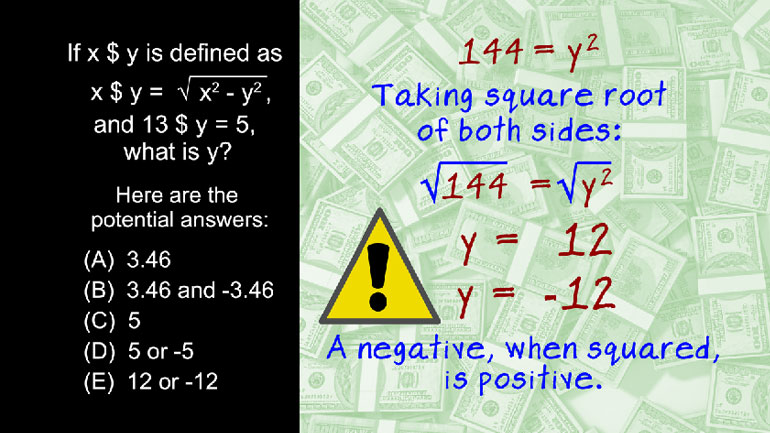
If x $ y is defined as x $ y equals the square root of x squared minus y squared,
- 00:18
and 13 $ y = 5, what is y?
- 00:22
And here are the potential answers…
Full Transcript
- 00:26
Is it just us are you seeing dollar signs?
- 00:29
So we're given that x $ y equals the square root of x squared minus y squared.
- 00:34
So, 13 $ y is the square root of 13 squared minus y squared.
- 00:43
The given information tells us that 13 $ y equals 5.
- 00:47
This can be written as the equation square root of 13 squared minus y squared equals 5.
- 00:53
This gives us square root of 169 minus y squared equals 5.
- 00:59
Let’s get rid of the square root by squaring both sides.
- 01:02
This gives us 169 minus y squared equals 25.
- 01:07
We add y squared to both sides, then subtract 25 from both sides to get 144 equals y squared.
- 01:14
Now we can take the square root of 144 to get y equals 12.
- 01:18
BUT…we have to remember that negative 12 is also an answer, since a negative, when
- 01:23
squared, is positive.
- 01:24
The answer is (E).
Related Videos
SAT Math 2.1 Geometry and Measurement. What is the measure of angle z in terms of x and y?
In 2014, the unemployment rate of one county in California was 7%. In another county, the unemployment rate was 11%. Which of the following express...
Angela is making cookies for a bake sale. She expects each batch of her cookies to sell for $40. It costs her $10 to make one batch of cookies, and...