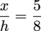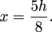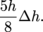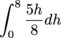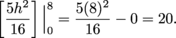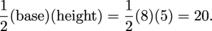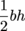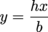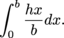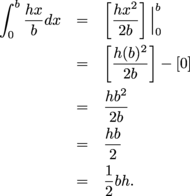We've made it. We survived years of seemingly endless lectures about trigonometry, proofs, and functions. Our 7th grade math teacher rattled off formula after formula for the areas and volumes of different polygons and solids. All we could do is sit there, listen, and memorize every formula we were given.
We were like power lines transporting the power—our geometry formula—from a power plant that produced the formula to the paper or project, the formula's final home. With calculus, we'll never make a memory error about a length, area, or volume formula again. If we aren't sure, we can produce our own formula on the spot.
With our new found power, we'll begin our production slowly. Triangles are the simplest and most useful polygons we come across, so we'll derive the formula for the area of a triangle first.
Example 1
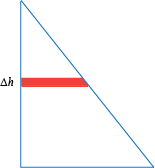
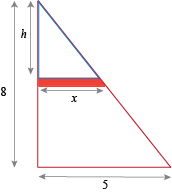
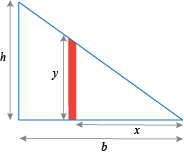
Find an integral expression for the area of the triangle, using slices as shown. Use h as the variable of integration.
|
Exercise 1
For each triangle,
(a) Find an integral expression for the area of the triangle using the slice and variable of integration indicated.
(b) Evaluate your integral and check that it agrees with the area you would find using the formula


Exercise 2
For each triangle,
(a) Find an integral expression for the area of the triangle using the slice and variable of integration indicated.
(b) Evaluate your integral and check that it agrees with the area you find using the formula


Exercise 3
For each triangle,
(a) Find an integral expression for the area of the triangle using the slice and variable of integration indicated.
(b) Evaluate your integral and check that it agrees with the area you find using the formula


Exercise 4
For each triangle,
(a) Find an integral expression for the area of the triangle using the slice and variable of integration indicated.
(b) Evaluate your integral and check that it agrees with the area you find using the formula


Exercise 5
For a triangle with base b and height h, use integration to derive the area formula

Use vertical slices with x for the variable of integration.