Since we're told to use h as the variable of integration, we have to take horizontal slices. These slices have thickness Δ h, since h changes a little bit from the top to the bottom of each slice. 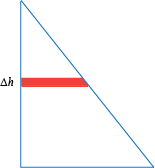
Remember that it's important to know what the variable h means. In this case h measures the distance from the top of the triangle to the slice. Call the length of the slice x. To find x, we use the fact that the blue and the pink triangles are similar triangles. 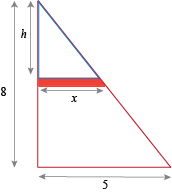
We can see from the similar triangles that 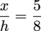
so 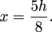
This means the area of the slice is 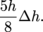
Since the triangle has height 8, the variable h ranges from 0 to 8. The slice at h = 0 is at the top of the triangle, and the slice at h = 8 is at the bottom of the triangle. The integral 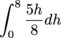
gives the area of the triangle. When we evaluate the integral, we get 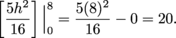
This is reassuring, because we know the area of this triangle is 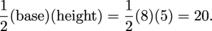
It should be clear that we could replace the numbers with variable parameters for base and height to get the area formula directly. With our new found super power to generate area formulas, we could move on to other geometries right away. But we want to get some more practice with this simplest polygon first. Since we already know the area formula for a triangle, we will use these problems to practice - using similar triangles,
- keeping track of what the variable of integration is,
- slicing every-which-way, and
- slicing a shape that isn't positioned on the coordinate axes.
|