Think you’ve got your head wrapped around Area, Volume, and Arc Length? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. Let R be the region bounded by the y-axis, the line y = 9, and the graph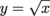 
. Which of the following integrals gives the volume of the region whose base is the region R and whose slices perpendicular to the x-axis are semicircles?
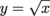. Which of the following integrals gives the volume of the region whose base is the region R and whose slices perpendicular to the x-axis are semicircles?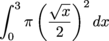 
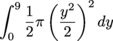
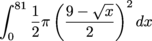
Q. Let R be the region bounded by the line y = 5 and the curve y = x2 + 1. What is the volume of the solid whose base is R and whose cross-sections perpendicular to the y-axis are squares?
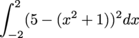
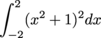
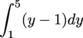
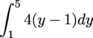
Q. The volume of a cone with base radius 6 and height 10 is found using horizontal slices as shown. Which integral best represents the volume of the cone?
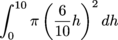
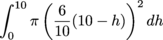
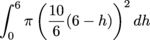
Q. What is the volume of the solid obtained by rotating the region graphed below around the x-axis?
(Write an integral for the volume, and evaluate your integral)
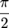
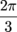
π
Q. The region bounded by the graphs y = x2, x = 2, and the x-axis is rotated around the line y = -2. Which of the following integrals gives the volume of the resulting solid, using the washer method?
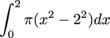
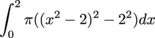
Q. The region bounded by the graphs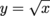 
and y = x is rotated around the x-axis. What is the volume of the resulting solid, using the washer method?
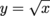and y = x is rotated around the x-axis. What is the volume of the resulting solid, using the washer method?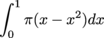 
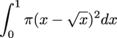
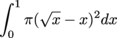
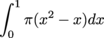
Q. Let R be the region bounded by the graph y = x2, the line x = 2, and the x-axis. What is the volume of the solid obtained when R is rotated around the line x = 0?
Use the washer method.
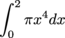
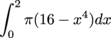
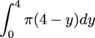
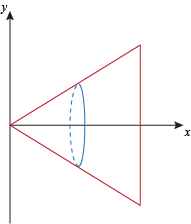
Q. Which picture would be most helpful for the following problem?
"Rotate the region bounded by the line y = x, the line x = 1, and the x-axis around the line x = 0. Use the shell method to find the volume of the resulting solid."
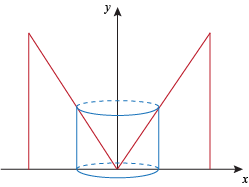
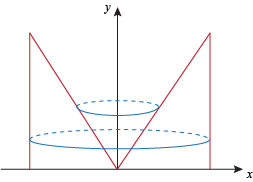
Q. Let R be the region bounded by the line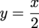 
and the curve 
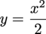. Rotate the region R around the x-axis.
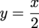and the curve 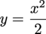. Rotate the region R around the x-axis.Which integral best represents the volume of the resulting solid, as found using the shell method?
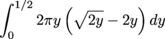
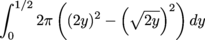
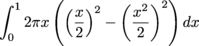
Q. A region R is rotated around the line y = 1 to generate a solid. Which of the following statements is true?
If we use the washer method to find the volume of R, the integral will be with respect to x. If we use the shell method to find the volume of R, the integral will be with respect to x.
If we use the washer method to find the volume of R, the integral will be with respect to x. If we use the shell method to find the volume of R, the integral will be with respect to y.
If we use the washer method to find the volume of R, the integral will be with respect to y. If we use the shell method to find the volume of R, the integral will be with respect to x.
If we use the washer method to find the volume of R, the integral will be with respect to y. If we use the shell method to find the volume of R, the integral will be with respect to y.