Unbalanced Forces
As we learned in the introduction to Newton’s Second Law and witnessed with orbital motion and the Universal Gravitation Law, an unbalanced force leads to acceleration. And, as we saw in projectile motion, sometimes that force and acceleration occur in only one of two dimensions of motion.
In the previous cases we studied, we only applied forces in one direction. It’s time to add up multi-dimensional forces. But don’t worry: we’ll take it one two-dimensional step at a time.
Friction is a force that opposes motion and acts on any object sliding or rolling on the surface of another object. Even air resistance is a type of friction. It exists because of molecular differences in the two surfaces at a microscopic level. If we had a perfectly polished glass sliding along another slab of perfectly polished glass, they could slide along one another without any friction to take energy away from their shared groove thing.
“Frictionless” is only possible in theory because “perfectly polished” is possible only in theory. In reality there will always be tiny bumps on the surface of our glass that will latch onto other tiny bumps that resist the object’s overall motion.
Like the normal force, friction is a contact force. Unlike the normal force, its magnitude is determined by how resistant two surfaces are to being rubbed against each other. In fact, it’s related to the magnitude of the normal force: Ff = μN, where μ is the coefficient of friction, determined by the two materials present. There are tables of these coefficients, but they can also be calculated from knowing the size of the normal force, N, and the size of the friction force, Ff.
To further complicate matters, friction has two sub-types: static friction and kinetic friction.
Static friction is the friction that opposes motion of sliding or rubbing. To overcome static friction, a force larger than the force static friction has to be present. However, once the two objects start moving kinetic friction is present. Rubbing a balloon on hair requires the presence of kinetic friction, come to think of it.
Static friction and kinetic friction have different coefficients for the same two materials. For example with glass on glass the coefficient of static friction may be μs = 0.9 while the coefficient of kinetic (moving) friction is μk = 0.3. These coefficients are unitless and calculable for any surface combination such as rubber on asphalt by utilizing force diagrams.
Even without calculation, we can get a feel for the size of static friction in comparison with kinetic friction by slowing increasing the amount of force we put on an object. Once we overcome static friction, it’s easier to push because the force to keep the velocity steady is smaller than before. Go ahead, try it, we know you want to.
Chew on this one for a minute: does a car rely on static friction or kinetic friction between tires and road?
The correct answer is static friction: the rubber on road isn’t slipping, it’s gripping. As the tire spins the rubber doesn’t slide, but if it did, we’d have skid marks and kinetic friction.
Next, let’s look at a force diagram involving static friction. These particular forces are balanced such that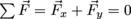
, which equation means the sum of all the forces is equal to the forces added in x and y direction separately and then combined with the Pythagorean Theorem. Any balanced forces still sum to zero, though.
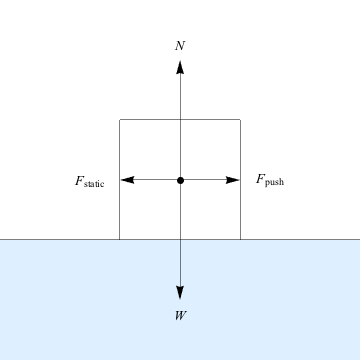
Here we have a block sitting on a rough surface even though someone’s pushing it, which creates static friction between the block and the surface itself. Friction always acts in the direction opposite to the motion. In this case, the normal force and the weight balance each other out, and the push is the direction of intended motion.
Note that no motion occurs because W = N and Fstatic = Fpush. The forces are all balanced. Static friction requires no motion, remember?
For the sake of argument, let’s assume that the push is equal to the maximum possible force from static friction before the object begins to slide, though if the push were less than the maximum force of static friction, the forces would still be balanced, because the static friction force would oppose it equally. If we know the magnitude of the pushing force, we could calculate the coefficient through Fstatic = Fpush = μsN = μsW.
If the pushing force increases beyond the maximum possible value of static friction, F = μsN, then the force of static friction turns into the force of kinetic friction, which is a smaller force vector, resulting in smaller coefficient.
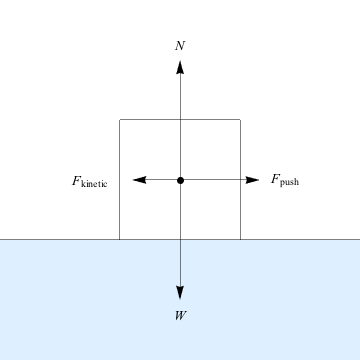
Now, as we sum up all our forces, the forces are only balanced vertically with W = N or Fy = N – W = 0. Same difference. In the x-direction, we’ve got Fx = Fpush – Fkinetic = ma. We can make more substitutions, turning the equation into Fpush – μkN = ma, then to Fpush – μkW = ma. We can reduce this further into Fpush – μkmg = ma. Putting forces in terms of weight mg is generally helpful. Everyone knows how to find weight.
Knowing weight, we can find friction. That may be true in more “weigh” than one, weight being the sticky topic that it is. Make like Phileas Fogg from Around the World in Eighty Days, and we quote:
It’s easier to see this if we draw another free-body diagram. For the inclined plane problem, we like to set our Cartesian coordinates origin on the center of mass so that the axes are aligned with the incline’s angle. Let’s have a look at the diagram below:
In the above diagram, we’ve tilted the coordinate axis with the incline since most of the forces involved are parallel or perpendicular to the incline. This means the only vector we need to worry about splitting into our new, not-horizontal-or-vertical x- and y- components is the force W, which falls vertically downward at an angle θ to the new coordinate system. With this trick, we can also say that we only have motion in the x-direction. Trust us; it’s simpler this way.
The diagram already broke down W into its new components: in our x-direction, we have W sin θ, and in the y-direction balancing out the normal force we have W cos θ. Trigonometry is an incline’s best friend.
Let’s try this, shall we?
We write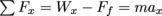
, while in the y-direction, we have 
. Note that it doesn’t matter which direction we call positive in either case. Here, we’ve chosen the direction the block will accelerate down the ramp as positive.
So far, so good. It would seem we’re building a great recipe on how to solve these infamous inclined plane problems. Next we can make substitutions, knowing what Wx, Wy, and Ff are. This force of friction is kinetic, because the ramp is sliding down already, so Ff = μkN.
Finding N from the y-direction, we get N = Wy = W cos θ = mg cos θ. If we knew the values of mass and angle, we could get a numeric value for N, but let’s leave it symbolic, just because. It’s a physics thing.
With the normal force to hand, we’re prepared to make an additional substitution within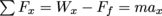
. Follow along: max = Wx – Ff = W sin θ – μkN = mg sin θ – μkmg cos θ. Whew!
Let’s rewrite that: max = mg sin θ – μkmg cos θ. See what we can do now? All terms have m, so it cancels out leaving ax = g sin θ – μk g cos θ. If we really wanted to simplify as much as mathematically possible, we could write ax = g(sin θ – μk cos θ).
Imagine if we had left the x- and y-axes in their usual locations, what would have happened? We’d have had to deal with components of both acceleration and friction in both x and y directions! By choosing to define the axes we did, the acceleration and friction was limited to one dimension. Therefore we had balanced forces in the other dimension. This let us find relationships between forces, and solve the problem. And just like that, we’ve found the acceleration of the block. Or whatever that rectangle stands for.
When we talk about inclines, there are several possible setups: we could be trying to figure out how friction balances gravity and makes a block sit still, or we might be interested in finding out how hard we have to push to slide a block up a hill, or we might be trying to calculate how quickly a block will slide down the slope and if we have enough time to get out of the way if our friend happens to let go. The possibilities are endless, but a free-body diagram is always the place to start.
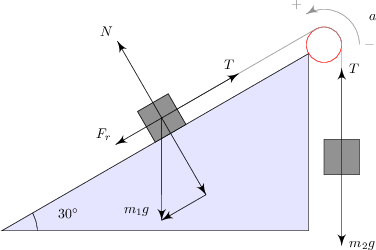
Another variation uses tension from a string or wire instead of a push. Just label it T and carry on as usual. A problem has a pulley system? Tension is there. Here’s an example of a two-for-one diagram, related by tension because tension is the same all along a string or wire. Otherwise, this problem is eerily similar to the one we already did. Give it a try!
Be careful that the right kind of friction makes it into the diagram: “Static” for stationary, “kinetic” for movement.
Include every force acting on the object on the diagram. Separate out x- and y-forces. Balanced forces add to zero, while unbalanced forces equal
.
In the previous cases we studied, we only applied forces in one direction. It’s time to add up multi-dimensional forces. But don’t worry: we’ll take it one two-dimensional step at a time.
Friction
Before we start to study two-dimensional motion in earnest, we need to know about friction, like really know about it.Friction is a force that opposes motion and acts on any object sliding or rolling on the surface of another object. Even air resistance is a type of friction. It exists because of molecular differences in the two surfaces at a microscopic level. If we had a perfectly polished glass sliding along another slab of perfectly polished glass, they could slide along one another without any friction to take energy away from their shared groove thing.
“Frictionless” is only possible in theory because “perfectly polished” is possible only in theory. In reality there will always be tiny bumps on the surface of our glass that will latch onto other tiny bumps that resist the object’s overall motion.
Like the normal force, friction is a contact force. Unlike the normal force, its magnitude is determined by how resistant two surfaces are to being rubbed against each other. In fact, it’s related to the magnitude of the normal force: Ff = μN, where μ is the coefficient of friction, determined by the two materials present. There are tables of these coefficients, but they can also be calculated from knowing the size of the normal force, N, and the size of the friction force, Ff.
To further complicate matters, friction has two sub-types: static friction and kinetic friction.
Static friction is the friction that opposes motion of sliding or rubbing. To overcome static friction, a force larger than the force static friction has to be present. However, once the two objects start moving kinetic friction is present. Rubbing a balloon on hair requires the presence of kinetic friction, come to think of it.
Static friction and kinetic friction have different coefficients for the same two materials. For example with glass on glass the coefficient of static friction may be μs = 0.9 while the coefficient of kinetic (moving) friction is μk = 0.3. These coefficients are unitless and calculable for any surface combination such as rubber on asphalt by utilizing force diagrams.
Even without calculation, we can get a feel for the size of static friction in comparison with kinetic friction by slowing increasing the amount of force we put on an object. Once we overcome static friction, it’s easier to push because the force to keep the velocity steady is smaller than before. Go ahead, try it, we know you want to.
Chew on this one for a minute: does a car rely on static friction or kinetic friction between tires and road?
The correct answer is static friction: the rubber on road isn’t slipping, it’s gripping. As the tire spins the rubber doesn’t slide, but if it did, we’d have skid marks and kinetic friction.
Next, let’s look at a force diagram involving static friction. These particular forces are balanced such that
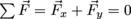, which equation means the sum of all the forces is equal to the forces added in x and y direction separately and then combined with the Pythagorean Theorem. Any balanced forces still sum to zero, though.Here we have a block sitting on a rough surface even though someone’s pushing it, which creates static friction between the block and the surface itself. Friction always acts in the direction opposite to the motion. In this case, the normal force and the weight balance each other out, and the push is the direction of intended motion.
Note that no motion occurs because W = N and Fstatic = Fpush. The forces are all balanced. Static friction requires no motion, remember?
For the sake of argument, let’s assume that the push is equal to the maximum possible force from static friction before the object begins to slide, though if the push were less than the maximum force of static friction, the forces would still be balanced, because the static friction force would oppose it equally. If we know the magnitude of the pushing force, we could calculate the coefficient through Fstatic = Fpush = μsN = μsW.
If the pushing force increases beyond the maximum possible value of static friction, F = μsN, then the force of static friction turns into the force of kinetic friction, which is a smaller force vector, resulting in smaller coefficient.
Now, as we sum up all our forces, the forces are only balanced vertically with W = N or Fy = N – W = 0. Same difference. In the x-direction, we’ve got Fx = Fpush – Fkinetic = ma. We can make more substitutions, turning the equation into Fpush – μkN = ma, then to Fpush – μkW = ma. We can reduce this further into Fpush – μkmg = ma. Putting forces in terms of weight mg is generally helpful. Everyone knows how to find weight.
Knowing weight, we can find friction. That may be true in more “weigh” than one, weight being the sticky topic that it is. Make like Phileas Fogg from Around the World in Eighty Days, and we quote:
He lived alone, and so to speak, outside of every social relation; and as he knew that in this world account must be taken of friction, and that friction retards, he never rubbed against anybody.
Inclines
In physics-speak, an incline is a ramp. Unlike Galileo’s experiment with as little friction as possible, we’ll continue to talk about forces as objects slide, rather than roll. The force of gravity is there too, as usual, and the normal force as well, but they won’t cancel each other out on an incline: the normal force won’t point opposite of gravity when the surface tilts.It’s easier to see this if we draw another free-body diagram. For the inclined plane problem, we like to set our Cartesian coordinates origin on the center of mass so that the axes are aligned with the incline’s angle. Let’s have a look at the diagram below:
In the above diagram, we’ve tilted the coordinate axis with the incline since most of the forces involved are parallel or perpendicular to the incline. This means the only vector we need to worry about splitting into our new, not-horizontal-or-vertical x- and y- components is the force W, which falls vertically downward at an angle θ to the new coordinate system. With this trick, we can also say that we only have motion in the x-direction. Trust us; it’s simpler this way.
The diagram already broke down W into its new components: in our x-direction, we have W sin θ, and in the y-direction balancing out the normal force we have W cos θ. Trigonometry is an incline’s best friend.
Let’s try this, shall we?
We write
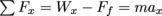, while in the y-direction, we have . Note that it doesn’t matter which direction we call positive in either case. Here, we’ve chosen the direction the block will accelerate down the ramp as positive.So far, so good. It would seem we’re building a great recipe on how to solve these infamous inclined plane problems. Next we can make substitutions, knowing what Wx, Wy, and Ff are. This force of friction is kinetic, because the ramp is sliding down already, so Ff = μkN.
Finding N from the y-direction, we get N = Wy = W cos θ = mg cos θ. If we knew the values of mass and angle, we could get a numeric value for N, but let’s leave it symbolic, just because. It’s a physics thing.
With the normal force to hand, we’re prepared to make an additional substitution within
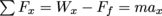. Follow along: max = Wx – Ff = W sin θ – μkN = mg sin θ – μkmg cos θ. Whew!Let’s rewrite that: max = mg sin θ – μkmg cos θ. See what we can do now? All terms have m, so it cancels out leaving ax = g sin θ – μk g cos θ. If we really wanted to simplify as much as mathematically possible, we could write ax = g(sin θ – μk cos θ).
Imagine if we had left the x- and y-axes in their usual locations, what would have happened? We’d have had to deal with components of both acceleration and friction in both x and y directions! By choosing to define the axes we did, the acceleration and friction was limited to one dimension. Therefore we had balanced forces in the other dimension. This let us find relationships between forces, and solve the problem. And just like that, we’ve found the acceleration of the block. Or whatever that rectangle stands for.
When we talk about inclines, there are several possible setups: we could be trying to figure out how friction balances gravity and makes a block sit still, or we might be interested in finding out how hard we have to push to slide a block up a hill, or we might be trying to calculate how quickly a block will slide down the slope and if we have enough time to get out of the way if our friend happens to let go. The possibilities are endless, but a free-body diagram is always the place to start.
Another variation uses tension from a string or wire instead of a push. Just label it T and carry on as usual. A problem has a pulley system? Tension is there. Here’s an example of a two-for-one diagram, related by tension because tension is the same all along a string or wire. Otherwise, this problem is eerily similar to the one we already did. Give it a try!
Common Mistakes
Always, no, never omit friction on a free-body diagram. If there’s acceleration and a contact force, by which we mean a normal force, there’s friction.Be careful that the right kind of friction makes it into the diagram: “Static” for stationary, “kinetic” for movement.
Include every force acting on the object on the diagram. Separate out x- and y-forces. Balanced forces add to zero, while unbalanced forces equal
.